In the 1930s, John von Neumann and Oscar Morgenstern became the founders of a new and interesting branch of mathematics called "game theory". In the 1950s, the young mathematician John Nash became interested in this area. The theory of equilibrium became the subject of his dissertation, which he wrote at the age of 21. Thus was born a new game strategy called "Nash Equilibrium", which won the Nobel Prize many years later - in 1994.
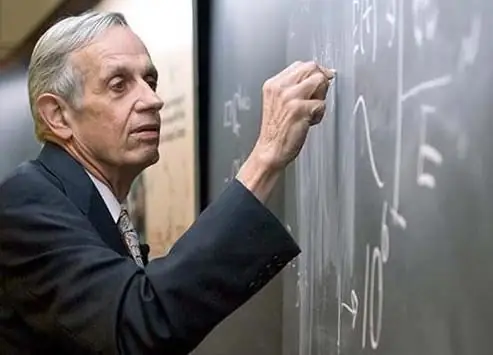
The long gap between writing a dissertation and general recognition has become a test for a mathematician. Genius without recognition resulted in serious mental disorders, but John Nash was able to solve this problem thanks to his excellent logical mind. His Nash Equilibrium theory won a Nobel Prize and his life was filmed in Beautiful mind.
Briefly about game theory
Since the Nash equilibrium theory explains the behavior of people in the conditions of interaction, it is worth considering the basic concepts of game theory.
Game theory studies the behavior of participants (agents) in terms of interaction with each other like a game, when the outcome depends on the decision and behavior of several people. The participant makes decisions based on his predictions about the behavior of others, which is called the game strategy.
There is also a dominant strategy in which the participant gets the best result for any behavior of other participants. This is the player's best win-win strategy.
Prisoner's dilemma and scientific breakthrough
Prisoner's dilemma is a case of a game where participants are forced to make rational decisions, achieving a common goal in the face of a conflict of alternatives. The question is which of these options he will choose, realizing personal and general interest, as well as the impossibility of getting both. The players seem to be imprisoned in a tough game environment, which sometimes makes them think very productively.

This dilemma was explored by the American mathematician John Nash. The balance he struck was revolutionary in its own way. Especially brightly this new thought influenced the opinion of economists about how market players make choices, taking into account the interests of others, with close interaction and intersection of interests.
It is best to study game theory through concrete examples, since this mathematical discipline itself is not dryly theoretical.
Prisoner's dilemma example
Example, two people committed a robbery, fell into the hands of the police and are being interrogated in separate cells. At the same time, police officers offer each participant favorable conditions under which he will be released if he testifies against his partner. Each ofcriminals have the following set of strategies that he will consider:
- Both testify at the same time and get 2.5 years in prison.
- Both are silent at the same time and receive 1 year each, because in this case the evidence base of their guilt will be small.
- One testifies and is released, while the other is silent and gets 5 years in prison.
Obviously, the outcome of the case depends on the decision of both participants, but they cannot agree, because they are sitting in different cells. The conflict of their personal interests in the struggle for a common interest is also clearly visible. Each of the prisoners has two options for action and 4 options for outcomes.
Chain of logical inferences
So, offender A is considering the following options:
- I am silent and my partner is silent - we will both get 1 year in prison.
- I turn in my partner and he turns me in - we both get 2.5 years in prison.
- I am silent, and my partner betrays me - I will get 5 years in prison, and he will be free.
- I hand over my partner, but he is silent - I get freedom, and he gets 5 years in prison.
Let's give a matrix of possible solutions and outcomes for clarity.
Table of possible outcomes of the prisoner's dilemma.
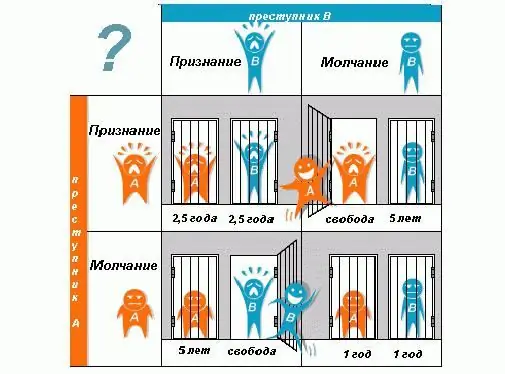
The question is, what will each contestant choose?
"Be silent, you can't speak" or "You can't be silent, you can't speak"
To understand the participant's choice, you need to go through the chain of his thoughts. Following the reasoning of criminal A: if I remain silent and my partner remains silent, we will receive a minimum term (1 year), but II don't know how he will behave. If he testifies against me, then it's better for me to testify, otherwise I can sit down for 5 years. I'd rather sit down for 2.5 years than 5 years. If he keeps silent, then all the more I need to testify, because that way I will get my freedom. Participant B.

It is not hard to see that the dominant strategy for each of the perpetrators is to testify. The optimal point of this game comes when both criminals testify and receive their "prize" - 2.5 years in prison. Nash game theory calls this equilibrium.
Non-optimal optimal Nash solution
The revolutionary nature of the Nashian view is that such an equilibrium is not optimal when considering the individual participant and his self-interest. After all, the best option is to remain silent and go free.
Nash equilibrium is a point of convergence of interests, where each participant chooses the option that is optimal for him only if other participants choose a certain strategy.
Considering the option when both criminals are silent and receive only 1 year, we can call it a Pareto-optimal option. However, it is possible only if the criminals could agree in advance. But even this would not guarantee this outcome, since the temptation to retreat from the agreement and avoid punishment is great. The lack of complete trust in each other and the danger of getting 5 years forced to choose the option with recognition. Reflect on what participants will adhere tooption with silence, acting in concert, is simply irrational. Such a conclusion can be drawn if we study the Nash equilibrium. Examples only prove you right.
Selfish or rational
The Nash Equilibrium Theory yielded startling conclusions that disproved the principles that existed before. For example, Adam Smith considered the behavior of each of the participants as completely selfish, which brought the system into balance. This theory was called the “invisible hand of the market.”
John Nash saw that if all participants act in their own interests, this will never lead to an optimal group result. Given that rational thinking is inherent in each participant, the choice offered by the Nash equilibrium strategy is more likely.
Purely male experiment
A prime example is the blonde paradox game, which, while seemingly out of place, is a clear illustration of how Nash game theory works.
In this game you need to imagine that a company of free guys came to a bar. Nearby is a company of girls, one of which is preferable to others, say a blonde. How do guys act to get the best girlfriend for themselves?

So, the reasoning of the guys: if everyone starts to get acquainted with the blonde, then, most likely, no one will get it, then her friends will not want to get acquainted. Nobody wants to be the second fallback. But if the boys choose to avoidblonde, then the probability for each of the guys to find a good girlfriend among the girls is high.
The Nash equilibrium situation is not optimal for guys, because, pursuing only their own selfish interests, everyone would choose the blonde. It can be seen that the pursuit of only selfish interests will be tantamount to the collapse of group interests. Nash equilibrium will mean that each guy acts in his own interests, which are in contact with the interests of the whole group. This is not the best option for everyone personally, but the best for everyone, based on the overall strategy for success.
Our whole life is a game
Decision making in the real world is very much like a game where you expect certain rational behaviors from other participants as well. In business, at work, in a team, in a company, and even in relationships with the opposite sex. From big deals to ordinary life situations, everything obeys one law or another.

Of course, the above game situations with criminals and a bar are just excellent illustrations that demonstrate the Nash equilibrium. Examples of such dilemmas very often arise in the real market, and this works especially in cases where two monopolists control the market.
Mixed Strategies
Often we are involved in not one, but several games at once. Choosing one of the options in one game, guided by a rational strategy, but you end up in another game. After a few rational decisions, you may find that your result is not to your liking. Whattake?
Let's consider two types of strategy:
- Pure strategy is the participant's behavior, which comes from thinking about the possible behavior of other participants.
- Mixed strategy or random strategy is the alternation of pure strategies at random or the choice of a pure strategy with a certain probability. This strategy is also called randomized.

Considering this behavior, we get a new look at the Nash equilibrium. If earlier it was said that the player chooses a strategy once, then another behavior can be imagined. It can be assumed that the players choose a strategy randomly with a certain probability. Games that cannot find Nash equilibria in pure strategies always have them in mixed strategies.
Nash equilibrium in mixed strategies is called mixed equilibrium. This is an equilibrium where each participant chooses the optimal frequency of choosing his strategies, provided that other participants choose their strategies with a given frequency.
Pen alties and mixed strategy
An example of a mixed strategy can be found in the game of football. The best illustration of a mixed strategy is perhaps a pen alty shootout. So, we have a goalkeeper who can only jump into one corner, and a player who will take the pen alty.
So, if the first time the player chooses the strategy to shoot to the left corner, and the goalkeeper also falls into this corner and catches the ball, how can things develop the second time around? If the playerwill hit in the opposite corner, this is most likely too obvious, but hitting in the same corner is no less obvious. Therefore, both the goalkeeper and the kicker have no choice but to rely on random selection.
Thus, by alternating a random choice with a certain pure strategy, the player and the goalkeeper try to get the maximum result.






