The article describes the role of statistics as a science. The concept of kurtosis and its use in science are considered.
Statistics. Basic concept
Statistics is the basic derivative of mathematical science. This subject belongs to a number of social disciplines that are aimed at forming a picture of the worldview and a competent analysis of events in students.
Statistics studies all kinds of processes and events in people's lives, highlights their patterns and presents everything in the form of short statistical reports. Such science is socially useful and requires constant improvement. Excess - what is it? This is a basic concept in graphical statistics that helps determine the correctness of perfect calculus. Kurtosis should not have a strong deviation.
Statistics allows you to satisfy people's need for reliable information about a particular phenomenon, event, and so on. Determining the dynamics of life factors, their decline, stagnation or growth - this is exactly what this science does.
In the modern world, statistics occupies one of the main places in the scientific arena. Let's consider the concept of "kurtosis". What is a statistical goal and observation? Where are these concepts used? Read more about all this inarticle.
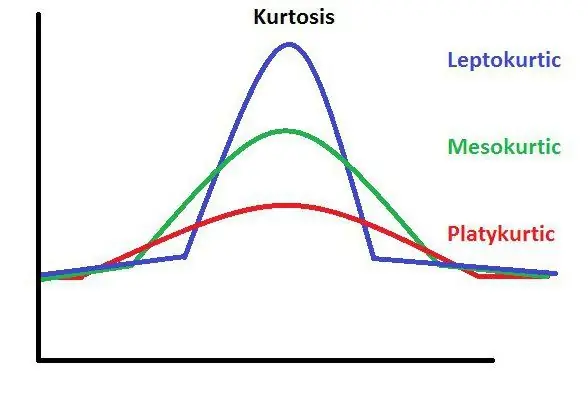
What is kurtosis in statistics?
Kurtosis is a statistical concept that represents the sharpness of each peak in a distribution graph. There is a special formula for its exact calculation.
Expectation states that for a more even distribution of statistical data, kurtosis must be equal to a positive number. Literally, this concept means a certain deviation from the norm and further abnormal development or functioning of the statistical system.
Excessive expression of kurtosis on a statistical graph may indicate an incorrect study or errors in the original data of the graph. Such a concept is scalar in nature, which means that the final calculation should result in a number that will not contain variables or auxiliary functions. This is what kurtosis looks like.
What is symmetric and asymmetric distribution
Kurtosis, which is related to the distribution of the symmetrical type, is equal to zero. This can be clearly shown with a graph. The asymmetric graph shows a number that is non-zero. This is how kurtosis is designated.
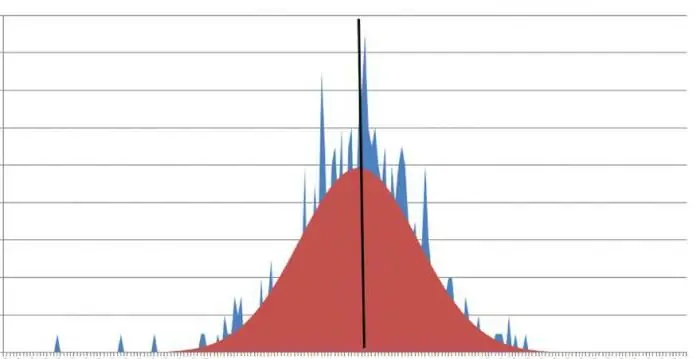
What is the sharpness of a peak? The graph, which has sharp peaks, indicates that the kurtosis value is a number less than zero. Often there are situations when a given concept cannot be expressed by a separate value. In this case, it approaches minus or plus infinity.






